A singular point of an algebraic curve is a point where the curve has "nasty" behavior such as a cusp or a point of self-intersection (when the underlying field 
is taken as the reals). More formally, a point 
on a curve 
is singular if the 
and 
partial derivatives of 
are both zero at the point 
. (If the field 
is not the reals or complex numbers, then the partial derivative is computed formally using the usual rules of calculus.)
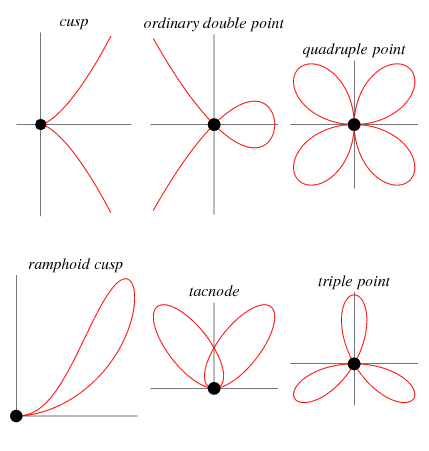
The following table gives some representative named curves that have various types of singular points at their origin.
| singularity | curve | equation |
| acnode |  | |
| cusp | cusp curve |  |
| crunode | cardioid |  |
| quadruple point | quadrifolium |  |
| ramphoid cusp | keratoid cusp |  |
| tacnode | capricornoid |  |
| triple point | trifolium |  |
Consider the following two examples. For the curve
 |
the cusp at (0, 0) is a singular point. For the curve
 |
is a nonsingular point and this curve is nonsingular.
Singular points are sometimes known as singularities, and vice versa.