The derivative of the function f(x) at the point
is given and
denoted by
Some Basic Derivatives
In the table below, u,v, and w are functions of the variable x. a, b, c, and n are constants (with some restrictions whenever they apply).
designate the natural logarithmic
function and e the natural base for . Recall that 
.
Chain Rule
The last formula
is known as the Chain Rule formula. It may be rewritten as
Another similar formula is given by
Derivative of the Inverse Function
The inverse of the function y(x) is the function x(y), we have
Derivative of Trigonometric Functions and their Inverses
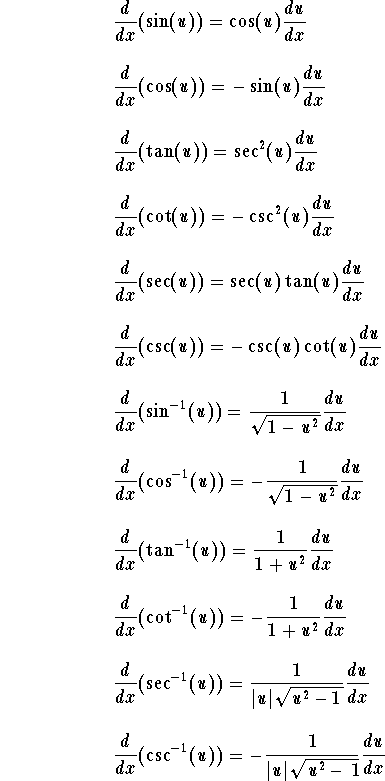
Recall the definitions of the trigonometric functions
Derivative of the Exponential and Logarithmic functions
Recall the definition of the logarithm function with base a > 0 (with
):
Derivative of the Hyperbolic functions and their Inverses
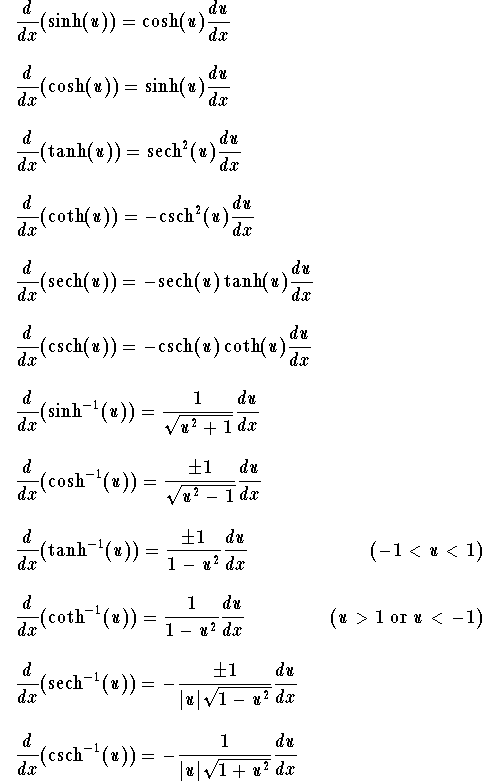
Recall the definitions of the trigonometric functions
Higher Order Derivatives
Let y = f(x). We have:
In some books, the following notation for higher derivatives is also used:
Higher Derivative Formula for the Product: Leibniz Formula
where
are
the binomial coefficients. For example, we have
