region R bounded by f, y = 0, x = a, and x = b is revolved about the y-axis, it generates a solid S, as shown in Fig. 1.2.
We wish to find the volume V of S. If we use the slice method as discussed in typical slice will be
perpendicular to the y-axis, the integration will be along the y-axis, the increment will be dy, and the integrand will be a
function of y. That entails solving the equation y = f(x) for x to get an equation of the form x = g( y). That task may be easy,
or hard, or impossible.
So in this section we're going to investigate another
approach, one that doesn't require solving the equation y = f(x) for
x. Let x be an arbitrary point in [a, b], as shown in Fig. 1.1. Erect a thin vertical rectangle at x of width dx and height
x. Let x be an arbitrary point in [a, b], as shown in Fig. 1.1. Erect a thin vertical rectangle at x of width dx and height
 |
Fig. 1.1The colored region is revolved about the y-axis. |
 |
Fig. 1.2The Solid Of Revolution And A Cylindrical Shell. |
 |
Fig. 1.3The cylindrical shell is reproduced here for clarity. |
f(x). The rectangle is gray
colored. When the region R
is revolved about the y-axis,
the vertical line segment at x
sweeps
out a cylindrical cross section, and the rectangle sweeps out a cylindrical shell, as shown gray colored in Fig. 1.2.
Note that the rectangular strip is parallel to the y-axis, which is the axis of revolution, and the cylindrical shell has its
axis along the axis of revolution. Let dV be the volume of the shell. The volume V of the solid S can be approximated by
the sum of the differential volume elements of such shells. Each shell in Fig. 1.2 corresponds to a rectangle in Fig. 1.1.
The rectangles run from a to b. Thus so do the shells. Consequently V is the integral of dV from x = a to x = b. Hence
the integration will be along the x-axis and the integrand will be a function of x (an expression involving f(x), as is
the case for the slice method; This shows that we won't have to solve the
equation y = f(x) for x. Now let's find the volume V. The cylindrical shell is reproduced in Fig. 1.3. Its volume dV is:
out a cylindrical cross section, and the rectangle sweeps out a cylindrical shell, as shown gray colored in Fig. 1.2.
Note that the rectangular strip is parallel to the y-axis, which is the axis of revolution, and the cylindrical shell has its
axis along the axis of revolution. Let dV be the volume of the shell. The volume V of the solid S can be approximated by
the sum of the differential volume elements of such shells. Each shell in Fig. 1.2 corresponds to a rectangle in Fig. 1.1.
The rectangles run from a to b. Thus so do the shells. Consequently V is the integral of dV from x = a to x = b. Hence
the integration will be along the x-axis and the integrand will be a function of x (an expression involving f(x), as is
the case for the slice method; This shows that we won't have to solve the
equation y = f(x) for x. Now let's find the volume V. The cylindrical shell is reproduced in Fig. 1.3. Its volume dV is:

This approach of finding the volume of revolution by using
cylindrical shells is called, well, the method of cylindrical
shells. For the sake of simplicity, it's also called the shell method.
shells. For the sake of simplicity, it's also called the shell method.
A solid generated by revolving a disk about an axis that is
on its plane and external to it is called a torus (a
doughnut-shaped solid). In we've found the volume of the torus using the slice
method. The torus is shown in Fig. 1.4. Find its volume using the shell method.
doughnut-shaped solid). In we've found the volume of the torus using the slice
method. The torus is shown in Fig. 1.4. Find its volume using the shell method.
 |
Fig. 1.4
A Torus.
|
Solution
 |
Fig. 1.5
The torus can be generated by
revolving this disk about the
y-axis. |

EOS
Remarks 1.1
i. The shell method gives the same result as does the
slice method.
ii. Instead of a rectangle, we simply draw a strip,
and call it a rectangle and treat it as such when finding the volume of
the generated cylindrical shell. This is because drawing a strip is simpler than drawing a rectangle and there's no harm
in doing so.
the generated cylindrical shell. This is because drawing a strip is simpler than drawing a rectangle and there's no harm
in doing so.
iii. The axis of revolution is the y-axis.
iv. The rectangle is parallel to the axis of
revolution.

vi. The rectangular strip is perpendicular to the x-axis. As a consequence the
increment is dx. Hence
the integration is
along the x-axis and we must express both the radius and the height of the shell in terms of x.
along the x-axis and we must express both the radius and the height of the shell in terms of x.
Example 2.1
In we used the slice method to find the volume of the solid
generated by revolving the plane region
bounded by y = x2 and y = 3 about the line y = –1. Now use the shell method to find that volume.
bounded by y = x2 and y = 3 about the line y = –1. Now use the shell method to find that volume.
Solution
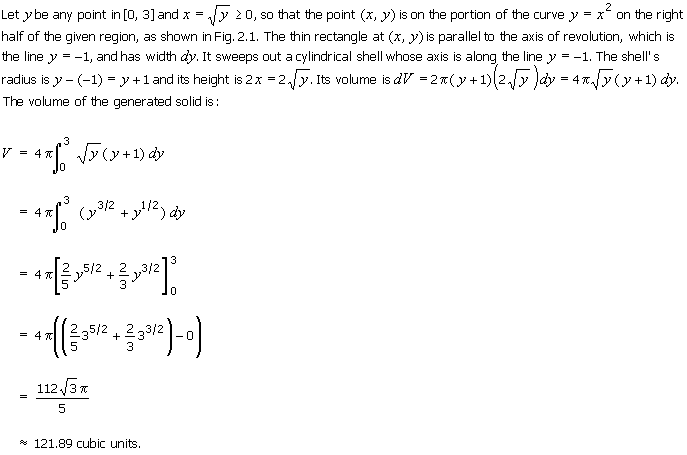
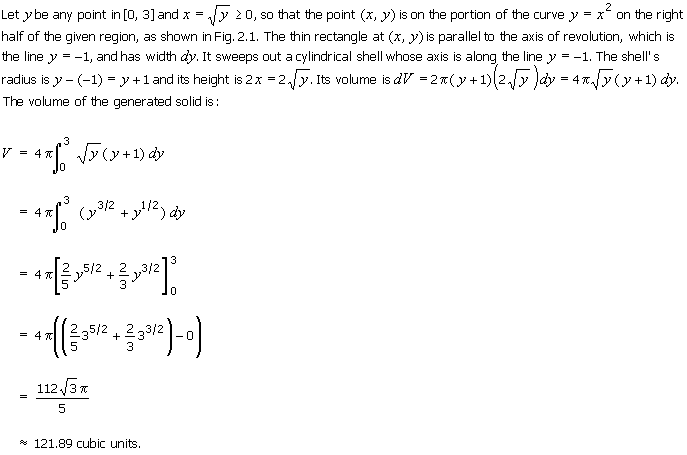
 |
Fig. 2.1Plane region bounded by y = x2 and y = 3 is revolved about line y = –1. |
EOS
Remarks 2.1
i. The shell method gives the same result as does the
slice method.
ii. The shell method can also be employed when the
axis of revolution doesn't coincide with a coordinate axis.
iii. The axis of revolution is parallel to the x-axis. The axis of revolution
is horizontal, while in the case for the torus in
Example 1.1 above it's vertical.
Example 1.1 above it's vertical.
iv. The rectangle is parallel to the axis of
revolution.

vi. The rectangular strip is perpendicular to the y-axis. Consequently the
increment is dy, the
integration is along the
y-axis, and we must express both the radius and the height of the shell in terms of y.
y-axis, and we must express both the radius and the height of the shell in terms of y.