Let a particle travel a distance 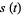 as a function of time
as a function of time 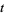 (here,
(here, 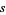 can be thought of as the arc length of the curve traced out by the particle). The speed (the scalar norm of the vector velocity) is then given by
can be thought of as the arc length of the curve traced out by the particle). The speed (the scalar norm of the vector velocity) is then given by
(1)
|
The acceleration is defined as the time derivative of the velocity, so the scalar acceleration is given by
(2)
| |||
(3)
| |||
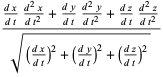 |
(4)
| ||
(5)
| |||
(6)
|
The vector acceleration is given by
(7)
| |||
(8)
| |||
(9)
|
where 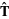 is the unit tangent vector,
is the unit tangent vector, 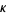 the curvature,
the curvature,  the arc length, and
the arc length, and 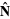 the unit normal vector.
the unit normal vector.
Let a particle move along a straight line so that the positions at times 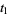 ,
, 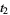 , and
, and 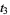 are
are 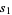 ,
, 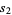 , and
, and 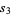 , respectively. Then the particle is uniformly accelerated with acceleration
, respectively. Then the particle is uniformly accelerated with acceleration 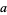 iff
iff
(10)
|
is a constant (Klamkin 1995, 1996).
Consider the measurement of acceleration in a rotating reference frame. Apply the rotation operator
(11)
|
twice to the radius vector 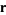 and suppress the body notation,
and suppress the body notation,
(12)
| |||
(13)
| |||
(14)
| |||
(15)
| |||
(16)
|
Grouping terms and using the definitions of the velocity 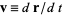 and angular velocity
and angular velocity 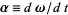 gives the expression
gives the expression
(17)
|
Now, we can identify the expression as consisting of three terms
(18)
| |||
(19)
| |||
(20)
|
a "body" acceleration, centrifugal acceleration, and Coriolis acceleration. Using these definitions finally gives
(21)
|
where the fourth term will vanish in a uniformly rotating frame of reference (i.e., 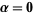 ). The centrifugal acceleration is familiar to riders of merry-go-rounds, and the Coriolis acceleration is responsible for the motions of hurricanes on Earth and necessitates large trajectory corrections for intercontinental ballistic missiles.
). The centrifugal acceleration is familiar to riders of merry-go-rounds, and the Coriolis acceleration is responsible for the motions of hurricanes on Earth and necessitates large trajectory corrections for intercontinental ballistic missiles.